Jump to content

File:Fourier transform time and frequency domains (small).gif

From mediawiki.org

Fourier_transform_time_and_frequency_domains_(small).gif (300 × 240 pixels, file size: 265 KB, MIME type: image/gif, looped, 93 frames, 37 s)

Description
English: A visualization of the relationship between the time domain and the frequency domain of a function, based on its Fourier transform.

The Fourier transform takes an input function f (in red) in the "time domain" and converts it into a new function f-hat (in blue) in the "frequency domain".

In other words, the original function can be thought of as being "amplitude given time", and the Fourier transform of the function is "amplitude given frequency".

Shown here, a simple 6-component approximation of the square wave is decomposed (exactly, for simplicity) into 6 sine waves. These component frequencies show as very sharp peaks in the frequency domain of the function, shown as the blue graph.
Date
Source Own work
Author Lucas V. Barbosa
Permission
(Reusing this file)
Public domain I, the copyright holder of this work, release this work into the public domain. This applies worldwide.
In some countries this may not be legally possible; if so:
I grant anyone the right to use this work for any purpose, without any conditions, unless such conditions are required by law.
Other versions

Higher resolution:

(separate files were necessary due to the awfulness of MediaWiki's thumbnail generator)

Captions

Add a one-line explanation of what this file represents

Items portrayed in this file

depicts

inception

23 February 2013

data size

271,468 byte

duration

36.65 second

height

240 pixel

width

300 pixel

media type

image/gif

checksum

189803ab9b4ef9b75c65518f182b13a59e4cee6b

determination method or standard: SHA-1

File history

Click on a date/time to view the file as it appeared at that time.

Date/TimeThumbnailDimensionsUserComment
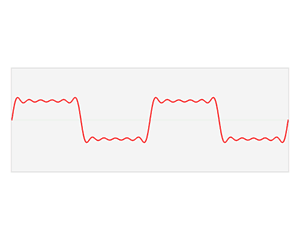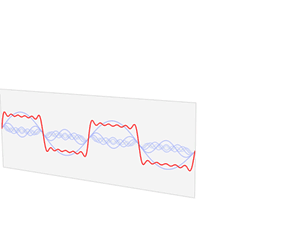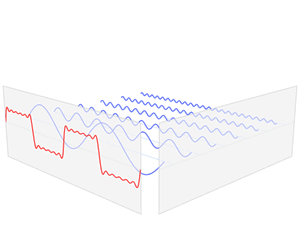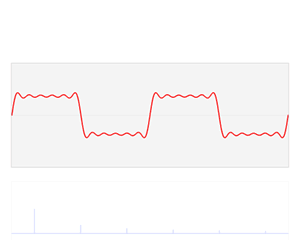
current20:44, 23 February 2013Thumbnail for version as of 20:44, 23 February 2013300 × 240 (265 KB)LucasVB{{Information |Description={{en|1=A visualization of the relationship between the time domain and the frequency domain of a function, based on its Fourier transform. The Fourier transform takes an input function f (in red) in the "time domain" and con...

The following page uses this file:

Global file usage

The following other wikis use this file:

View more global usage of this file.

Retrieved from "https://www.mediawiki.org/wiki/File:Fourier_transform_time_and_frequency_domains_(small).gif"